2つのベクトルの内積からなす角を求めるポイント：ベクトル

2016/3/1 2016/3/13 平面上のベクトル, 数学, 数学B

2つのベクトルの内積を利用して、なす角を求める問題です。
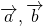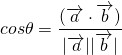2つのベクトル $\overrightarrow{a}, \overrightarrow{b}$ がなす角 $\theta$ に関して、 $cos\theta=\dfrac{(\overrightarrow{a}\cdot \overrightarrow{b})}{|\overrightarrow{a} ||\overrightarrow{b} |}$ が成り立つことを利用します。

スポンサーリンク

なす角を求める問題

次の2つのベクトルのなす角 $\theta$ を求めなさい。
$\overrightarrow{a}=(1,~\sqrt[]{\mathstrut 3}), \overrightarrow{b}=(3, ~\sqrt[]{\mathstrut 3})$

2つのベクトルの内積からなす角を求める解法の手順

2つのベクトルの大きさをそれぞれ求めます。
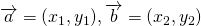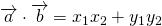$\overrightarrow{a}=(x_1,y_1 ), \overrightarrow{b}=(x_2,y_2 )$ のとき、 $\overrightarrow{a } \cdot \overrightarrow{b}=x_1 x_2+y_1 y_2$ となることから内積を求めます。
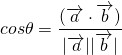なす角 $\theta$ に関して $cos\theta=\dfrac{(\overrightarrow{a}\cdot \overrightarrow{b})}{|\overrightarrow{a} ||\overrightarrow{b} |}$ が成り立つことから 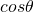$cos\theta$ を求めます。
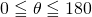$0 \leqq \theta \leqq 180$ の範囲で、求められた 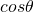$cos\theta$ の値をとる角 $\theta$ を求めます。

なす角を求める問題の解説

$\overrightarrow{a}と\overrightarrow{b}$ の大きさは、それぞれ

$|\overrightarrow{a} |=~\sqrt[]{\mathstrut (1^2+(~\sqrt[]{\mathstrut 3})^2 )}=2$

$| \overrightarrow{b} |=~\sqrt[]{\mathstrut (3^2+(~\sqrt[]{\mathstrut 3})^2 )}=2~\sqrt[]{\mathstrut 3}$

となり、また、

$\overrightarrow{a} \cdot \overrightarrow{b}$

$=13+~\sqrt[]{\mathstrut 3}~\sqrt[]{\mathstrut 3}$

$=3+3$

$=6$

となります。よって、 $\overrightarrow{a}と\overrightarrow{b}$ のなす角を $\theta$ とすると、

$cos\theta=\dfrac{(\overrightarrow{a}\cdot \overrightarrow{b})}{|\overrightarrow{a} ||\overrightarrow{b} |}$

$=\dfrac{6}{(2 \times 2~\sqrt[]{\mathstrut 3})}$

$=\dfrac{3}{(2~\sqrt[]{\mathstrut 3})}$

$=\dfrac{~\sqrt[]{\mathstrut 3}}{2}$

となります。

2つのベクトルのなす角は0°以上180°以下となるので、この2つのベクトルのなす角は

$cos\theta=\dfrac{~\sqrt[]{\mathstrut 3}}{2}$

$\theta=60$

と求められます。

参考

数学1 チャート式　数研出版

関連記事

スポンサーリンク